算法设计与分析-Week1

发表于 | 更新于

Container With Most Water

题目描述

Given n non-negative integers a1, a2, …, an , where each represents a point at coordinate (i, ai). n vertical lines are drawn such that the two endpoints of line i is at (i, ai) and (i, 0). Find two lines, which together with x-axis forms a container, such that the container contains the most water.
Note: You may not slant the container and n is at least 2.

图1

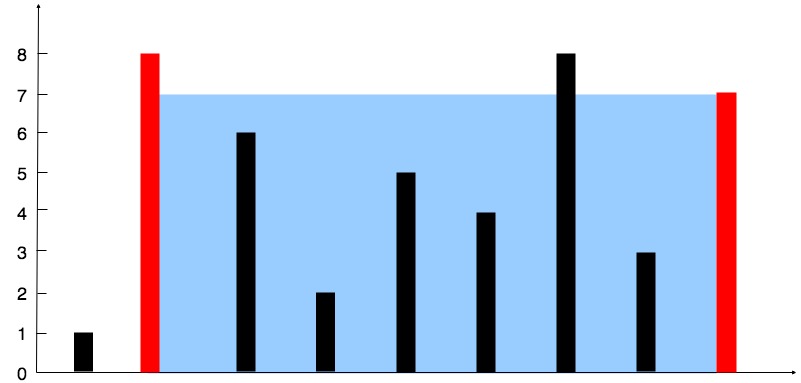
The above vertical lines are represented by array [1,8,6,2,5,4,8,3,7]. In this case, the max area of water (blue section) the container can contain is 49.
Example:

Input: [1,8,6,2,5,4,8,3,7]
Output: 49

解题思路

题目要求最大可装水的体积，可装水的体积取决于水桶两边的高以及底边的宽度，根据短板效应，可装水的高度不会超过两边的较短边的高度，因此可装水的体积=水桶两边的较短边的高度*底边的宽度。

解法一

暴力求解。将所有边两两组合并求体积，用两重循环即可解决，但时间复杂度为\(O(n^2)\)

解法二

先选取最左边和最右边两条边，记录此时的可装水的体积，由于该体积取决于两边的较短边，因此我们希望该较短边越长越好，于是我们将较短边替换成更内层的边，并计算新的可装水体积，与原来的体积作比较取较大值。最后当两边取到同一边时退出循环，得到最大可装水体积，时间复杂度为\(O(n)\)

源代码

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
class Solution {
public:
    int maxArea(vector<int>& height) {        

        int left = 0;
        int right = height.size() - 1;

        int max = containWater(height[left], height[right], right - left);
        while(left < right){
            if(height[left] < height[right]){
                left++;
                int temp = containWater(height[left], height[right], right - left);
                if(temp > max){
                    max = temp;
                }
            }
            else{
                right--;
                int temp = containWater(height[left], height[right], right - left);
                if(temp > max){
                    max = temp;
                }
            }
        }
        return max;
    }
    int containWater(int left, int right, int width){
        if(left < right) return left * width;
        else return right * width;
    }
};
0%